SCEC2021 Poster #151: Scaling of the solution in an elastodynamic rupture problem: an analytical proof

Poster #151, Fault and Rupture Mechanics (FARM)

Scaling of the solution in an elastodynamic rupture problem: an analytical proof

Baoning Wu, David D. Oglesby, Christodoulos Kyriakopoulos, & Jennifer M. Tarnowski

Poster Image:

Poster Presentation

2021 SCEC Annual Meeting, Poster #151, SCEC Contribution #11470 VIEW PDF

It has long been known that the solution of an elastodynamic earthquake rupture problem scales with some initial stress and friction parameters. For a dynamic rupture model with a slip-weakening friction law, if we scale the initial stress and slip weakening distance by the same numerical factor, the solution will be identical, except with the dynamic outputs (e.g., slip, slip rate, ground motion) being scaled by the same factor. Such an argument is widely applied in the non-dimensional analysis of dynamic rupture models, especially in early dynamic rupture models where model setup is simple. 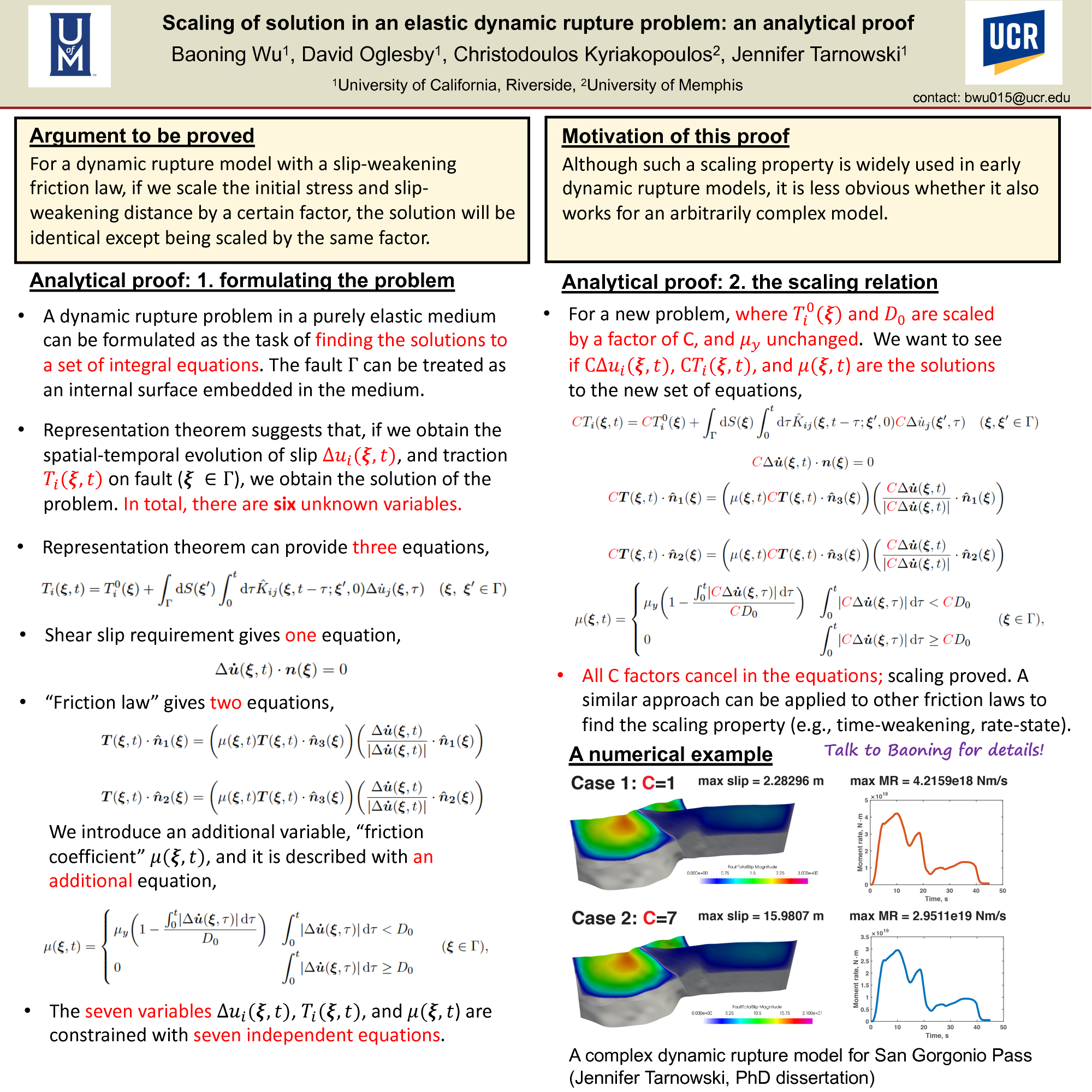However, a simple proof for this scaling property hasn’t been thoroughly documented to the best of o...ur knowledge. As dynamic rupture models become more complex recently, questions arise about whether such a scaling property can also be applied in a more complicated situation, especially among rookie dynamic rupture modelers. In this research, we show with an analytical proof that the scaling of the solution in an elastodynamic rupture model under certain frictional parameterizations should hold true in a general sense, no matter what the fault geometry, medium elastic property, or free surface conditions are. Beyond a slip-weakening friction law, our analysis can also be extended to elastodynamic rupture models with time-weakening friction law or rate-and-state friction law. We note that our analysis does not apply to anelastic media, and we suspect that an anelastic dynamic rupture model may not have such a scaling property.
SHOW MORE